Chemical Potential

If a small quantity of A, \( dn_A \) is added to a large quantity of a phase at a constant temperature and pressure, the size of the system will increase by \( dn_A \), and therefore the total Gibbs Free energy of the system will also change by a small amount dG. If dnA is small enough, dG will be proportional to the amount of A added. i.e., \[ dG \propto dn_{A} \] On Adding proportionality constant \( \mu_A \) \[ dG = \mu_A dn_A \] \( \mu_A \) : chemical potential of A in the phase, and is function of composition. Due to this, \( dn_A \) should be small enough so that the composition of the phase is not altered. Hence, the chemical potential of A is defined as,

\[ \Rightarrow \boxed{ \mu_A = \Bigg(\frac{dG}{dn_A}\Bigg)_{P,T, n_{j \neq i}} } \]

Hence, Chemical Potential \( \mu_A \) can be define as free energy per mole or partial molar free energy.

Calculation of Chemical Potential \( ( \mu ) \)

\( \mu_A \) and \( \mu_B \) can be obtained by extrapolating the tangent to the G curve to the sides of the molar Gibbs Free energy diagram Column. And finally, \[ G = \mu_A X_A + \mu_B X_B \] Now, \( X_A + X_B = 1 \Rightarrow dX_A = -dX_B \) \[ \frac{dG}{dX_B} = \mu_A \frac{dX_A}{dX_B} \Rightarrow \boxed{\frac{dG}{dX_B} = \mu_B - \mu_A}\]

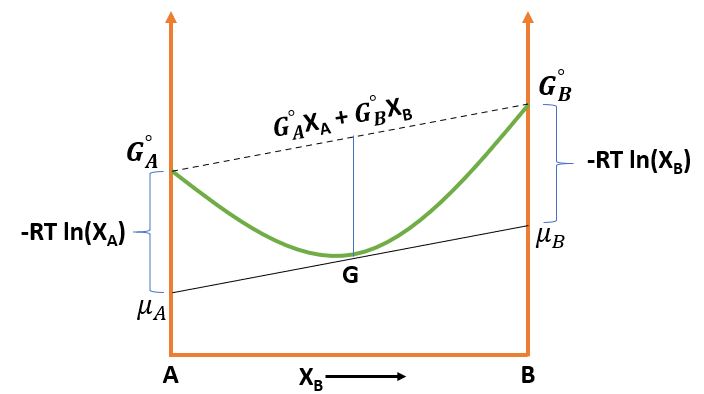
\[ \mu_A = G_A + RT \ln{X_A} \] \[ \mu_B = G_B + RT \ln{X_B} \]

Thermodynamic Activity

Activity (symbol \( \alpha \) ) is the measure of the effective concentration of a chemical species under non-ideal behaviour. Activity is a dimensionless quantity and the activity depends on the following factors:

• Temperature
• Pressure
• Composition of the mixture, etc.